Understanding Oscillators: Types, Working Principles and Real-World Applications

Oscillators are fundamental components in electronic circuits, generating periodic waveforms that serve as the backbone for various applications, from simple timing circuits to complex communication systems. By converting direct current (DC) into alternating current (AC), oscillators create repetitive signals that can be either sine, square or triangle waves, among others.

This article aims to provide a comprehensive overview of the different types of oscillators, how they work and where they are applied. Whether you’re an electronics hobbyist, an engineer or simply curious about how oscillators influence modern technology, this guide will offer valuable insights.

What is an Oscillator?

An oscillator is an electronic circuit that generates a continuous, repetitive signal, typically in the form of a sine, square, or triangle wave. Unlike amplifiers, which amplify input signals, oscillators do not require an external input signal to produce output; they rely on feedback within the circuit to sustain oscillation.

Oscillators are characterized by several key parameters, including frequency, amplitude, and stability. The frequency refers to how many times the waveform repeats per second, measured in Hertz (Hz). Amplitude indicates the peak value of the waveform, while stability refers to the oscillator’s ability to maintain a consistent frequency and amplitude over time, despite environmental changes.

The type of signal generated by an oscillator—whether sine, square, or triangle—depends on its design and application. Sine wave oscillators, for example, are often used in radio and audio applications due to their smooth, continuous waveform. Square wave oscillators are favored in digital circuits because of their sharp transitions between high and low states, making them ideal for clock generation and digital signal processing.

Working of Oscillator

The working of an oscillator hinges on the principle of positive feedback, where a portion of the output signal is fed back into the input to sustain the oscillation. The core components of an oscillator include an amplifier and a feedback network. The amplifier’s role is to boost the signal to a sufficient level, compensating for any energy losses in the circuit. This amplification ensures that the oscillations are maintained continuously. Meanwhile, the feedback network, composed of passive components such as resistors, capacitors, and inductors, filters and controls the feedback signal. For oscillations to be sustained, the feedback signal must be in phase with the original signal and have the necessary amplitude to reinforce it.

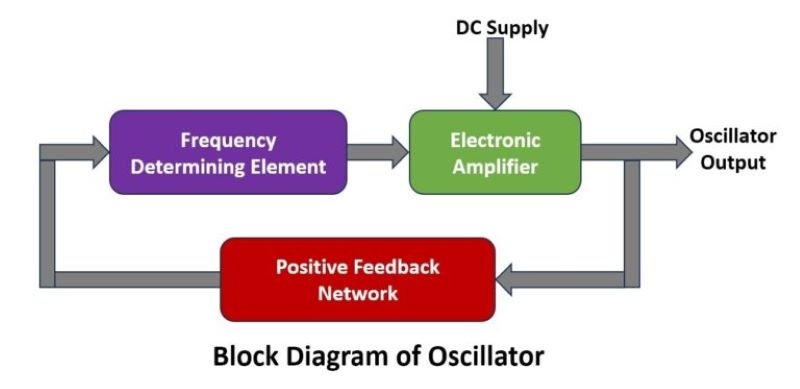
For an oscillator to function, it must meet the Barkhausen criterion, which specifies that the loop gain (product of the amplifier gain and feedback) must be exactly one (|Aβ| = 1), and the total phase shift around the loop must be either zero or an integer multiple of 360 degrees. The frequency of the output signal is determined by the components within the feedback network. For example, in an LC oscillator, the resonant frequency is set by the inductance (L) and capacitance (C) values, while in an RC oscillator, the frequency is governed by the resistor and capacitor values. This precise control over the frequency allows oscillators to generate stable and consistent waveforms used in various electronic applications.

Classification of Oscillators

Oscillators are broadly classified into two main categories: Linear (Harmonic) Oscillators and Non-Linear (Relaxation) Oscillators.

Linear (Harmonic) Oscillators

Linear oscillators, also known as harmonic oscillators, generate smooth, periodic waveforms, typically sine waves. These oscillators rely on the resonance of an LC (inductor-capacitor) circuit or the frequency-determining properties of a crystal to maintain oscillation. The key characteristic of linear oscillators is their ability to produce stable and low-distortion waveforms.

Types of Linear Oscillators:

• RC Oscillators: Utilize resistors and capacitors to determine the frequency of oscillation. They are commonly used in low-frequency applications like audio signal generation.
• LC Oscillators: Employ inductors and capacitors to create high-frequency oscillations, making them suitable for radio frequency (RF) applications.
• Crystal Oscillators: Use the mechanical resonance of a vibrating crystal (usually quartz) to produce a very stable frequency. These are widely used in precision timing applications, such as in clocks, watches, and microprocessors.

Non-Linear (Relaxation) Oscillators

Non-linear oscillators, or relaxation oscillators, generate waveforms that are not sinusoidal, such as square, sawtooth, or triangle waves. These oscillators operate by charging and discharging a capacitor through a resistor or other non-linear components, resulting in rapid transitions between different states. Relaxation oscillators are typically used in applications where precise waveform shapes are required, rather than frequency accuracy.

Types of Non-Linear Oscillators:

• Multivibrator Oscillators: These include astable, monostable, and bistable multivibrators. Astable multivibrators generate continuous square waves, monostable multivibrators produce single pulses, and bistable multivibrators act as flip-flops or memory elements in digital circuits.
• Sawtooth Oscillators: Produce a waveform that linearly rises and then sharply falls, resembling the teeth of a saw. These oscillators are commonly used in signal processing, function generators, and television scanning circuits.

Working Principles of Major Oscillator Types

RC Oscillators

RC oscillators generate oscillations using resistors (R) and capacitors (C) as their primary frequency-determining components. These oscillators are typically used in low-frequency applications and are valued for their simplicity and ease of design.

Explanation: An RC oscillator generally consists of an amplifier and a feedback network of resistors and capacitors. The feedback network shifts the phase of the output signal by 180 degrees, and the amplifier provides another 180-degree phase shift, resulting in the necessary 360-degree (or 0-degree) phase shift for sustained oscillations. The frequency of oscillation is determined by the values of the resistors and capacitors.

Common Designs:

• Phase Shift Oscillator: Uses multiple RC stages (usually three) to achieve the required 180-degree phase shift. The frequency of oscillation is given by f = ( 1 / 2πRC√6 ).
• Wien Bridge Oscillator: Utilizes a Wien bridge network in the feedback loop, consisting of a series RC circuit and a parallel RC circuit. This design is known for its stability and low distortion in generating sine waves. The frequency of oscillation is f = ( 1 / 2πRC).

LC Oscillators

LC oscillators use an inductor (L) and a capacitor (C) to form a resonant tank circuit. These oscillators are widely used in radio frequency (RF) applications because they can generate high-frequency signals with low phase noise.

Explanation: In an LC oscillator, the inductor and capacitor store energy alternately, creating oscillations at the circuit’s natural resonant frequency. The circuit typically includes a transistor or operational amplifier to sustain the oscillations by compensating for energy losses in the tank circuit.

Common Designs:

• Hartley Oscillator: Features an inductor with a tap or a pair of inductors in series and a capacitor in parallel. The frequency of oscillation is f = ( 1/ 2π√LeqC’ ) where, Leq​ is the equivalent inductance.
• Colpitts Oscillator: Uses a single inductor and a pair of capacitors in series to form the tank circuit. The frequency of oscillation is  f = ( 1 / 2π√L(C1C2)/(C1+C2) ).

Crystal Oscillators

Crystal oscillators utilize the mechanical resonance of a vibrating crystal, typically quartz, to generate highly stable oscillation frequencies. These oscillators are essential in applications requiring precise timing, such as in clocks, watches, and microprocessors.

Explanation: A crystal oscillator circuit includes a quartz crystal connected to an amplifier with feedback. The crystal’s piezoelectric properties cause it to vibrate at a specific frequency when subjected to an electric field. The circuit amplifies and sustains these vibrations, resulting in a stable output frequency determined by the crystal’s physical dimensions.

Application in Precise Frequency Generation: Crystal oscillators are unmatched in frequency stability and accuracy, making them the go-to choice for timing and synchronization in digital systems, telecommunications, and instrumentation.

Multivibrator Oscillators

Multivibrator oscillators are non-linear circuits that generate square waves and pulses. They are widely used in digital electronics, timing circuits, and waveform generation.

Explanation: Multivibrators consist of a pair of active devices (transistors or operational amplifiers) that switch states, producing a square wave or pulse output. The circuit’s configuration determines its operation mode: astable, monostable, or bistable.

Types:

• Astable Multivibrator: Continuously oscillates between two states, generating a square wave without any external triggering.
• Monostable Multivibrator: Generates a single pulse in response to an external trigger, returning to its stable state afterward.
• Bistable Multivibrator: Also known as a flip-flop, it has two stable states and can be toggled between them using external inputs.

Applications of Oscillators

Here are some common applications of oscillators are as follows:

1. Communication Systems

Oscillators are central to communication systems, where they generate carrier waves in transmitters and local oscillator signals in receivers. They enable frequency modulation (FM), amplitude modulation (AM), and phase modulation (PM) in wireless communication, allowing for the transmission and reception of data over various frequency bands.

2. Signal Generators

Oscillators are used in function generators and waveform synthesizers to produce a variety of waveforms, such as sine, square, and triangle waves. These waveforms are essential in testing and calibrating electronic equipment, as well as in signal processing applications.

3. Timing Circuits

Oscillators provide the clock signals necessary for synchronizing operations in digital circuits, such as microcontrollers, processors, and digital watches. They ensure that all parts of a system operate in harmony, maintaining precise timing for tasks and processes.

4. Power Conversion

In power electronics, oscillators drive the switching elements in power supplies, inverters, and converters. They enable the conversion of DC to AC power and the regulation of output voltage and frequency, which is crucial for efficient energy management in various applications.

5. Consumer Electronics

Oscillators are ubiquitous in consumer electronics, where they generate audio signals in devices like radios, televisions, and music players. They also contribute to video signal processing and synchronization in TVs and monitors, as well as clock generation in computers and gaming consoles.

Factors to Consider in Oscillator Design

• Frequency Stability: Ensuring that the oscillator maintains a consistent frequency despite variations in temperature, load, and supply voltage is crucial for applications requiring precision, such as communication systems and instrumentation.
• Power Consumption: Minimizing power consumption is essential in battery-powered devices, where energy efficiency directly impacts the device’s operational lifespan.
• Load Influence and Temperature Effects: The oscillator’s performance can be affected by the load it drives and changes in temperature. Designers must account for these factors to ensure reliable operation across different conditions.

Conclusion

Oscillators plays an imp. role in nearly every aspect of modern electronics, from communication systems and signal processing to timing circuits and power conversion. Understanding the different types of oscillators and their working principles is essential for selecting the right one for a given application.

As technology advances, the demand for more precise, stable, and efficient oscillators continues to grow. Innovations such as MEMS oscillators and integrated circuit designs are pushing the boundaries of what oscillators can achieve, promising even more exciting developments in the future.